Enhancement and Modulation of Near-Field Radiative Heat Transfer Between Nanoparticles by a Graphene-hBN Multilayer

Paper Information:
Kun Zhang, Bo Zhang, Zixue Luo, Jinlin Song, Qiang Cheng. Enhancement and modulation of near-field radiative heat transfer between nanoparticles by a graphene-hBN multilayer. International Journal of Heat and Mass Transfer, Volume 236, Part 1, January 2025, 126288.
Link to the paper

Research Background

In the macroscopic domain, radiative heat transfer (RHT) follows the Stefan-Boltzmann law and Planck’s law, which define the upper limit of radiative heat flux between two objects, known as the black-body limit. Due to the existence of evanescent waves, when two objects are separated by subwavelength distances, the radiative heat flux can exceed the black-body limit. At certain frequencies, anomalous electromagnetic responses such as surface plasmon polaritons (SPPs) and surface phonon polaritons (SPhPs) can further enhance RHT. Based on these characteristics, near-field radiative heat transfer (NFRHT) has shown potential applications in areas such as thermophotovoltaics, thermal management, thermal imaging, and thermal memory.

RHT between nanoparticles has been a focus of research. When the size of the nanoparticles is much smaller than the characteristic wavelength of thermal radiation, they can be modeled as dipoles in calculations. Using this approximation, researchers have discovered many new physical phenomena in RHT between nanoparticles, such as thermal splitting, thermal routing, thermal Hall effect, thermal superdiffusion, and negative differential thermal conductivity.

The NFRHT between two objects is closely related to the probability of photon tunneling and the number of channels involved in heat transfer. That is, by introducing additional channels or adjusting the photon tunneling probability, the RHT between two nanoparticles can be modulated. In nanoparticle integration, many-body interactions can either enhance or suppress NFRHT depending on the material and structural design. Similarly, in planar many-body systems, radiation heat flux can also be effectively modulated through appropriate design.

Interestingly, by introducing additional surface modes supported by plates, it is expected that the heat flow between nanoparticles can be regulated. When nanoparticles are placed on one side of a plate, remote super-Planck heat transfer can be defined using the system’s reflection Green’s function. If an intermediate plate exists between the nanoparticles, energy is transferred through transmitted evanescent fields. Specifically, when the intermediate structure supports weakly dissipative hyperbolic modes, the transmitted energy is significantly amplified. Previous research mainly focused on the performance of artificial metamaterials. However, as a naturally occurring hyperbolic material, hexagonal boron nitride (hBN) is also expected to achieve such enhancement. Additionally, graphene-supported SPPs can couple with the hyperbolic phonon polaritons (HPPs) of hBN, which helps increase the probability of photon tunneling.

When the graphene-hBN (G-hBN) heterostructure is extended to a multilayer, the stacked metal-dielectric structure can generate more efficient hyperbolic properties. The supported hybrid polaritons include surface plasmon-phonon polaritons (SPPPs) and hyperbolic plasmon-phonon polaritons (HPPPs), which can be flexibly tuned by adjusting the chemical potential of graphene and the thickness of hBN. In this paper, we investigate the NFRHT between nanoparticles when the G-hBN multilayer acts as an intermediate waveguide.

We first discuss how geometric parameters, such as the number of layers and multilayer thickness, affect the radiative heat flux density. By analyzing the energy transfer coefficient between nanoparticles mediated by the G-hBN multilayer, we reveal the physical mechanisms of enhanced RHT. We then demonstrate the possibility of dynamically modulating RHT by adjusting the chemical potential of graphene layers. Finally, we explore the impact of the outermost graphene layer on energy transfer.

Research Content

As shown in Figure 1, two nanoparticles are placed on opposite sides of the G-hBN multilayer film. The energy of the hot nanoparticles is transferred to the cold layer through the multilayer film. The coordinates of nanoparticles p1 and p2 with radius $r=5\text{nm}$ are (x1,y1,z1)(x_1, y_1, z_1)(x1​,y1​,z1​) and (x2,y2,z2)(x_2, y_2, z_2)(x2​,y2​,z2​), respectively. The multilayer film is composed of $N$ layers of hBN, each of thickness $t$, and $N+1$ layers of graphene.

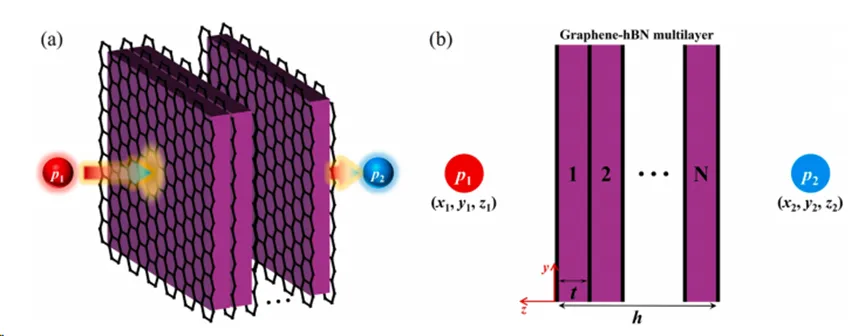
Figure 1:
(a) Schematic diagram of energy transfer between two nanoparticles through the G-hBN multilayer.
(b) Geometrical parameter schematic.

We consider the system shown in Figure 1, where the parameters are set as z1=50 nm,x1=x2,y1=y2z_1 = 50 \, \text{nm}, x_1 = x_2, y_1 = y_2z1​=50nm,x1​=x2​,y1​=y2​, and the distance between p2p_2p2​ and the multilayer is z1z_1z1​. The temperature of nanoparticle p1 is T1=310 KT_1 = 310 \, \text{K}T1​=310K and p2 is T2=Tm=300 KT_2 = T_m = 300 \, \text{K}T2​=Tm​=300K, ensuring that the power received by p2 comes only from the radiation of p1. To quantify the enhancement effect of the G-hBN multilayer on RHT, we define the enhancement factor η=P/P0\eta = P / P_0η=P/P0​, where the superscript 0 refers to the case with two nanoparticles in vacuum.

Figure 2 shows the relationship between the enhancement factor $\eta$ and the thickness $h$ and number of layers $N$ of the G-hBN multilayer. In the calculation, the thickness $h$ ranges from 0.1 to 10 μm, and the number of layers $N$ ranges from 1 to 20. As $h$ increases, $\eta$ initially increases and then decreases. When $h\approx 0.1\mu \text{m}$, the presence of the multilayer does not significantly improve RHT. When $h$ approaches 1 μm, the enhancement factor exceeds three orders of magnitude compared to the vacuum case. The enhancement factor increases with $N$, reaching its maximum value of 1722 at $h=0.9\mu \text{m}$ and $N=13$.

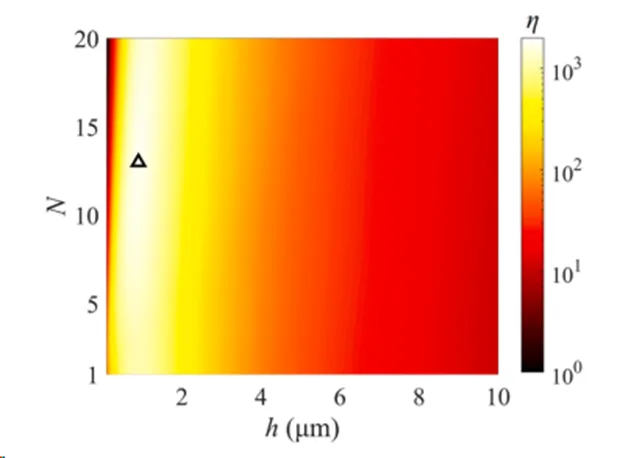
Figure 2:
Enhancement factor $\eta$ between two GSCS nanoparticles as a function of the G-hBN multilayer thickness $h$ and the number of layers $N$. Black triangle markers indicate the maximum $\eta$ values.

Conclusion and Outlook

In this study, we investigated the NFRHT between two GSCS nanoparticles placed on opposite sides of a G-hBN multilayer film. Through weakly dissipative hyperbolic modes, energy from hot nanoparticles can be effectively transferred to cold nanoparticles. The key conclusions are as follows:

1. When the thickness and number of layers of the G-hBN multilayer are appropriately chosen, the enhancement factor between the two nanoparticles can exceed 1000, primarily due to the coupling between the SPPs of graphene and the HPPs of hBN with the high-frequency localized surface resonances (LSRs) of the GSCS nanoparticles.
2. By adjusting the chemical potential of the graphene layers, the hybrid polaritons outside the hyperbolic region change significantly, resulting in tunable RHT between nanoparticles. For example, when the thickness is 0.5 μm, tuning the chemical potential can change the enhancement factor by hundreds of times.
3. We found that the enhancement factor of the G-hBN-G cell multilayer is approximately 1.63 times greater than that of the hBN-G-hBN cell multilayer, indicating the significant role of the outermost graphene layer in enhancing energy transfer in the G-hBN multilayer.